



How to TRISECT an angle. |

|
The ancient Greeks tried to do all their drawings just using a straight edge and a pair of compasses, but one of the things they found impossible was trisecting an angle - in other words dividing an angle into exactly three equal parts. That's because they had some very strict rules!
Over the last few years, hundreds of MM visitors have sent in their ideas for trisecting an angle. Sadly none of them worked but here we're going to show you a method that you'll find in the MM of Everything that does work BUT ... it cheats! And then we'll show you a brilliant method sent in from Joe Maddox in Florida USA. You can decide if Joe's method works or not!
 1/
1/
We need to start with any angle we like. The method we use should be able to trisect any angle, but if it can do angles between 30º and 90º then it can easily be adapted for any other angle. |
 2/
2/
You lie a ruler along one of the lines, and draw a line along the top. This is a slightly cheaty way of drawing a parallel line, but as you can easily draw parallel lines using straight edge and compasses, nobody can really moan yet. | |||
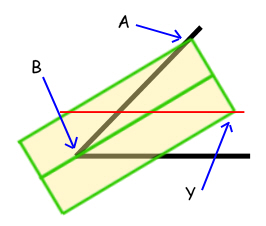 3/
3/
You now put two rulers together and lie them on your diagram as shown here. The middle of the two rulers lies on point B, The top corner lies on point A and the lower corner lies on the line you just drew at point Y. To get this right takes a bit of fiddling about ... and that DOES break the rules! |
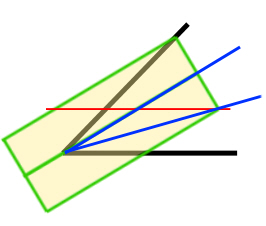 4/
4/
You now draw one line along the middle of the two rulers, and another line that comes from the corner and goes through the lower corner of the rulers. These lines TRISECT your angle exactly! | |||

| ||||
| ||||
The trouble is that the Greeks would NOT like part 3/ where two rulers get shuffled around until they fit on the diagram.
But now, here's another way of trisecting an angle that doesn't break the rules!
How to trisect an angle without cheating? Sent in by Joe Maddox, Ocala, Florida, USA | |||||||||||||||
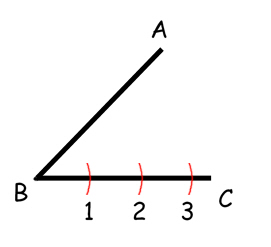 1/
1/
We can start with any angle in between about 30º and 90º. Here we've labelled the angle ABC. Using compasses measure off three equal sections along BC labelled 1,2,3. |
 2/
2/
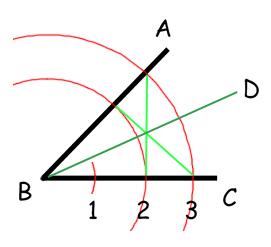
Draw two big arcs centred on B, one to pass through point 2 and the other to pass point 3. You now bisect the angle ABC. One way to do it is to draw in two lines between the points where the arcs cross the lines AB and BC, then draw the bisector BD through the point where these new lines cross. All nice and easy so far. | ||||||||||||||
 3/
3/
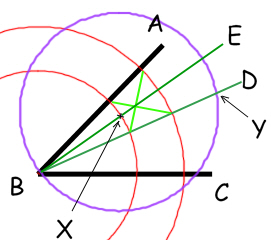
Now you bisect angle ABD. You can use cross lines again, and you end up drawing line BE. After that, you draw a circle. The centre point is X which is where BE crosses the "2" arc, and the circle goes through point B. (So the circle will have the same radius as the 2 arc.) The circle cuts BD at point Y. |
 4/
4/
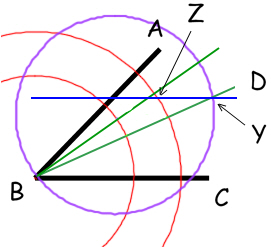
You now draw a line through Y parallel to BC. (If you don't know how to construct parallel lines with a straight edge and compasses, you'll find it in Savage Shapes. Sorry, we don't have space to tell you here!) This parallel line cuts the 3 arc at point Z. | ||||||||||||||
 5/
5/
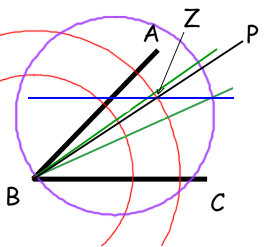
You draw a line from B that goes through Z to P. This line divides the angle in the ratio 1:2 ! |
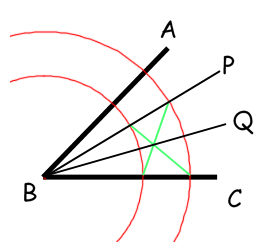 6/
6/
Finally you bisect the angle PBC and draw line BQ. The angle has been trisected perfectly ... or has it? | ||||||||||||||

| |||||||||||||||
| |||||||||||||||
If you enjoyed this page, find out how we SQUARED THE CIRCLE!